
Specifications
Weight: 45.52 carats
Dimensions: 25.60 x 21.78 x 12.00 mm
Color: Fancy Dark Grayish-Blue
Weight of Rough: Cut from French Blue
Origin: French Blue
Date Found: Cut in the early 1800’s
Current Location: Smithsonian Institution, Washington, D.C.
Details
The Hope diamond is the world’s second largest blue diamond, yet its provenance is shrouded in mystery and myth. Some have theorized that it is the recut form of a much larger stone, the French Blue, stolen during the French Revolution and recut to hide its identity. This study used computer modeling to show that it was possible that the Hope was cut from this stone. The controversy surrounding the Hope’s origin has been on-going ever since it appeared, and this project has added to the factual knowledge concerning this very important diamond.
The Hope diamond has a 200 year history. It appeared on the world scene around 1810 in a fully-cut form and was the world’s largest blue diamond up until just a few years ago. However, no references have ever been recorded of a piece of blue diamond rough having been found anywhere previous to its appearance. The Hope diamond’s source has been rumored to be a recut of another large blue diamond, the “French Blue”, sometimes referred to as the “Blue Diamond of the Crown.” This study used historical data and modern techniques to construct computer models of these two diamonds. A third diamond, the Tavernier Blue, was the parent stone of the French Blue, and it too was modeled to refine the French Blue model. These models were compared to see if it is reasonable, using knowledge of modern stone-cutting techniques and CAD, to expect that the Hope could have been cut from the French Blue. Additionally, if this geneology was possible, whether other stones made when recutting the 115.16 carat* Tavernier Blue to the 68.9 carat French Blue, or to the 45.52 carat Hope, as has been postulated by numerous sources.
*Note: References to weight are in new carats (0.2 gm/carat – new, 0.2053 gm/carat – old). The new carat was adopted in the late 1800’s, and reported stone weights prior to this period have been corrected to new carats to reflect this difference. New carats are used throughout this paper to avoid confusion. Weights of the three stones are as follows (old carats in parentheses); Hope – 45.52 (44.5), French Blue – 68.9 (67 1/8), Tavernier Blue – 115.16 (112 3/16 ).
Method
This study used computer-generated models of the French Blue (FB) and Hope diamonds to determine if the Hope could have been cut from the FB. Modeling of the FB was accomplished by using best available historical data (line drawings for shape and facet pattern, text for size) and modeling software. An additional stone, the Tavernier Blue (TB), is known to be the parent stone of the FB. It was also modeled as this allowed refinements to be made to the FB model to make it as accurate as possible. The model of the Hope was generated using digital pictures in combination with photomodeling and modeling software packages.
Photomodeling of the Hope required taking digital pictures of the unset diamond from all angles to capture the details concerning facet size, shape, and placement, girdle size and shape, and overall stone size and shape. Pictures from the top, bottom, and sides were taken from eight different rotational perspectives. Over 150 pictures were taken, and 27 were used to gather over 800 data points. These points were captured by the photomodeling software, then processed to output a stone diagram in CAD form.
The picture-taking process consisted of unmounting the Hope and placing it on a special cardboard grid. Discussions with the software developers of the photomodeling program revealed that the software corrected for spheroidicity of the lens, parallax, and other optical parameters that had to be considered to render an accurate computer reconstruction. To do this, the stone had to be placed on an accurate grid of known dimensions to validate the photographic data. After extensive conversations with the software developers, it was decided to manufacture a 1 cm grid on a thick piece of cardboard. The stone was centered on this grid for all pictures. Different photographic views were taken by rotating the cardboard.
A Canon A40 digital camera, with 2 megapixel resolution, was used to take one set of photographs. Another set was taken using a Nikon 990 camera with 3 megapixel resolution. Although a single camera would have been sufficient, a second camera was used so no single point of failure (camera malfunction, storage card erasure, etc) would destroy all the data. Two entirely different lighting conditions (incandescent and fluorescent) were used in two separate locations to further enhance data collection.
The Hope has a large culet facet that allows it to stand up on its own without any encumbering support structure that could hide important details from the camera. Each camera was mounted on a tripod, in turn, to take pictures of the stone. A series of dots were placed on the grid, and visible in each picture, to maintain a position reference as the cardboard and stone were rotated (Figure 1). Camera-to-stone distance was constant within each set of pictures to minimize scaling effects.
Once the pictures were acquired, they were downloaded into a computer and analyzed for usability. This involved looking at each one to determine if there was sufficient detail and clarity to make it useful. Lighting angle and intensity and depth of field issues ruled out many photos. Eventually, 27 photos were selected for data gathering.
Data gathering consisted of analyzing each photo and identifying every visible facet junction in that photo. Each junction was assigned its own identifying number, which would be maintained for all photos where the point could be identified. Additionally, each camera view was assigned an identity, and the visible data points were correlated to that view. The photomodeling software would track each of the points and the camera position, correct for optical parameters, and plot the position relationships of each point.
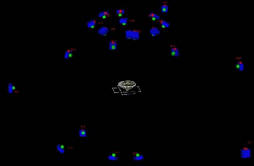
Once all pictures were entered, it performed a series of calculations to infer the position of each point relative to the others, and in so doing, build up a collection of all points. These were then gathered to generate a 3D model of the Hope.

Modeling of the French Blue (FB) was a little more problematic. Since the stone disappeared in 1792, no photos could be taken of it. However, a search of historical documents revealed line drawings of the stone made during its existence. One set of drawings, circa 1748, was made of the stone when it was set in an ornament called the “Order of the Golden Fleece”, showing the stone set in the ornament from both top and bottom (no side) views (Figure 2).
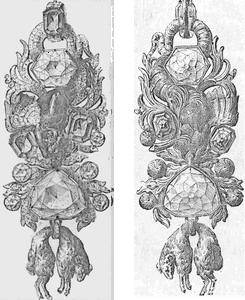

Dimensions of the stone were reported by Morel as being 31 x 24.81 x 12.78 mm and weighs 69.00 metric carats. The line drawings of each view were digitally transferred into the SolidWorks program. Using the program, a solid “box” of the necessary size and shape was generated, then slices were removed from this solid to emulate the grinding of facets. After many iterations of the slicing configuration, a 3D model of the stone was generated that matched the drawings.
This model of the FB was further refined by modeling the TB. It is a historical fact that the FB was cut from the 115.16 carat TB in the early 1670’s. The only picture of the TB are three line drawings from a mid-17th century book written by the stones European discoverer, Jean-Baptiste Tavernier, titled Six Voyages en Turquie, en Perse et aux Indes. The drawings show a top and bottom view, and one side view (Figure 4). It was decided to model the TB to further refine the FB model.
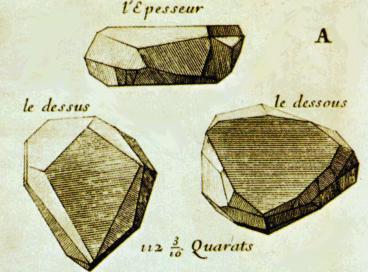
A model of the TB was created using the “upper” and “under” views. As published, these two views do not have the same outline, indicating that at least one was taken from an oblique perspective. Analysis of the picture Tavernier labels as “The Upper Part” revealed that some of the facets were oriented as if they were part of an original diamond crystal. This was corroborated by superimposing this picture on a computer-rendered perfect diamond crystal. This information shows that “The Upper Part” was drawn orthogonally, or nearly so, to the top surface of the stone, and the “The Under Part” was taken obliquely.
Modeling of the TB started by generating a theoretical solid with an outline replicating that of “The Upper Part” of Tavernier’s drawing, and a thickness corresponding to that of the FB. Setting the thickness to that of the FB was considered reasonable, as a diamond cutter would attempt to conserve as much material as possible when recutting, and could now reuse the two largest facets of the TB.
Using the photomodeling program, coupled with a knowledge of stone cutting, pieces were removed from the computer model to emulate facet size and shape depicted in the drawing of “The Upper Part”. Facet placement was iterated until the model surface replicated that in the drawing.
Once the top of the TB was complete, “The Under Part” was modeled using the same process. Now both views were modeled, but it became apparent that the third view, “The Thickness”, was not able to be resolved within the constraints dictated by the other two views. Additionally, there were some facets in the side view that are geometrically impossible. This is possibly the result of the artist adding lines thought to be on the surface when in face they were merely reflections, or losing surface lines in the reflections and so were not drawn. As a result, this view was disregarded for modeling purposes. After a cubic zirconia model of this stone was made, it became apparent that it would be very easy to incorrectly draw the side view.
Since Tavernier did not report dimensions, only weight (112 3/16 old carats), this model was initially dimensionless. Now the model was adjusted dimensionally using the photomodeling program, as it has the capability to change model dimensions to enclose a known weight if the density of the material is known. A value of 3.51 g/cc, a mid-range density value for diamond, was used. The program then calculated the required length and width to enclose a solid of this density to yield a weight of 115.16 new carats.
The first FB model was now further refined using the TB model. Since the FB was cut from the TB, the former had to fit inside the latter. Both models were then iterated within the known constraints of size, shape, and facet pattern until one fit inside the other.
Next, the photo-generated Hope model was superimposed on the FB model and any protrusions were noted. These were resolved by further iterating the FB model within historical constraints. These changes were then compared to the TB model for further iteration. Iterating the FB and TB models was considered necessary due to limited modeling information, and considered acceptable as long as all known physical constraints were still being met.
Once the computer models were complete, both wax and plastic models were made using a computer-driven milling machine. This tool generated solid, 3D models within a tolerance of 0.05 mm. These models were then used to compare the stones in a solid form.
Discussion
The Hope model was considered to be the most accurate of the three models due to it being constructed using modern techniques from the actual stone. Both the FB and TB models used in these comparisons were developed from line drawings of unknown accuracy. As a result, many models of these two stones could exist, yet still meet the historical physical descriptions.
A process of “forensic geometry” was used to build models of both the FB and TB. Descriptions of these stones are limited, consisting primarily of old line drawings and very limited text of their physical descriptions. Since there is no hard forensic evidence to compare the stones, forensic geometry was employed to build the most accurate models possible. This involved using all known physical attributes such as dimensions, weight, and facet pattern to develop models of them that satisfied these criteria.
Refinements to the models involved sliding the first model of the FB inside the first model of the TB. Areas of the FB that protruded from the TB model were noted. The models were then adjusted within known historical constraints to accommodate the protrusions, then the models were compared again. This process was repeated, with changes made to stay within the necessary constraints, until the FB fit inside the TB, as history declares that they must. Now the refined version of the FB could be compared to the Hope model.
The Hope model was considered the most accurate and one not to be adjusted. If it didn’t fit inside the updated version of the FB model, then the FB model was iterated until it did. These changes to the FB were then compared to the TB model. Both the FB and TB models were iterated until all three stones could nest one inside the other, with no overlap, and still maintain historical constraints. This method was used as the limited modeling information allowed many possible configurations of both stones.
Variations of the FB model do in fact support the case that the Hope could have been cut from the FB, and no model suggests the contrary. Placing the Hope model inside the French Blue model (Figure 5) indicates that it is possible that the FB is the parent stone of the Hope, as one fits inside the other.
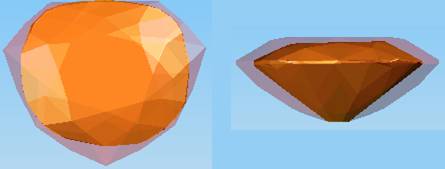
(Modeling by Steve Attaway)
Further evidence of a link between the Hope and the FB can be seen by looking at the shape of both stones. The shape of the Hope is that of an asymmetrical ellipse (Figure 1), a rather unusual form for a stone that was cut around 1800 in Europe, as it would have been cut for both symmetry and brilliance. But this begs the question “Why this shape?”
Cutting a symmetrical gem from a stone, whether a piece of rough out of the ground or recutting an existing stone, removes more material than if it were cut asymmetrically. Early Indian cutters recognized this, so they cut diamonds more for size rather than symmetry or brilliance. However. European tastes tended to the opposite. The Hope in its cut form was “discovered” in England, suggesting European cutting, but why would a European cutter cut such a rare stone slightly asymmetrical?
The computer models show that the Hope just barely fits inside the FB in its asymmetric form. If it was symmetrical, it would not fit unless it were a smaller stone. It is reasonable to assume that anyone stealing a major diamond, the biggest blue diamond in the world, would try to hide the crime, yet maximize their gain from that crime. Recutting the FB disguises its origin, especially if the shape is changed, as there were no definitive tests available at the time to link it to another stone, even if recut. Unfortunately, this results in a loss of weight, and therefore, value. The Hope’s shape is a reasonable compromise between the conflicting criteria of symmetry and retention of weight, while satisfying European demands of brilliance.
Superimposing the two models shows further evidence that the Hope could be a recut FB. The Hope is cut in a pattern known as an oval brilliant, a very standard cut even today. However, it has two additional facets on its pavilion along the girdle. Unfortunately, due to nondisclosure constraints enforced by the Smithsonian, a picture of these extra facets cannot be displayed here. However, this is an unusual facet arrangement, especially for such a notable diamond. Why would there be two extra facets on a stone that is otherwise cut in a normal arrangement? Comparing the position of these two facets on the Hope with the alignment within the FB suggests that these are original facets from the FB. Reusing facets would be done to keep as much of the original stone as possible.
The modeling also shows that the Hope fits inside the FB one, and only one, way. The tolerances are so tight that there is no room for any other configuration. This is to be expected in if the FB were recut into the Hope.
If the Hope were cut from the FB, are there sister stones to the Hope made from the leftover material of this process? Several diamonds have been suggested as having this scenario as their provenance, most notably the Russian Stickpin and long-lost Brunswick Blue diamonds. The Russian Stickpin diamond is a lozenge-shaped stone of 7.6 carats and currently residing in the State Diamond Fund of the Russian Federation, Inv. No. AF-8. The Brunswick Blue disappeared over 100 years ago and reportedly weighed 13.75 carats. Could these two be sister-stones to the Hope?
If the FB were recut into the Hope, there would be a loss of approximately 24 carats (69-45=24). Could there be recovered material from this operation to create stones of any significant size? After all, both the Russian Stickpin and Brusnwick Blue are significantly smaller than this loss. The answer is no. The tolerances between the two models show that the thickest piece to be removed, assuming absolutely perfect cleaving or sawing, is approximately 2 mm thick. Neither the Stickpin or Brunswick Blue diamond meet this criterion, and so are definitely not related to this cutting process. The biggest stone that could result, if a round brilliant, would be less than 3 mm in diameter and would weight about 0.25 carats, assuming perfect conditions in either sawing or cleaving. As a result, if the FB were cut into the Hope, there are no sister-stones greater than 0.25 carats.
Could the Brunswick Blue or Russian Stickpin be created when the TB was cut into the FB? When this occurred, there was a loss of approximately 46 carats (115-69=46). This is a loss slightly larger than a stone the size of the Hope. It is reasonable to assume that some of this loss was recovered in pieces large enough to create a stone of 7.6 carats, or even 13 carats.
Plastic models of the TB, FB, and Hope were milled out using a computer-controlled milling machine, accurate to a tolerance of 0.05 mm. These models were then used to compare the stones in solid form.
The plastic model of the TB was put into liquid rubber, then the rubber allowed to cure and harden. The rubber was carefully cut open and the plastic model removed, leaving a cavity the size and shape of the TB. Next, the FB model was placed inside this void in the orientation as shown by the computer modeling. Then hot wax was injected, filling the space between the plastic model and the rubber. When opened, the plastic FB was contained within a wax TB. The wax was cut away, resulting in a 3D solid emulating the amount of waste resulting from the TB-to-FB cutting process.
Using this process, it was possible to separate two irregularly shaped pieces of approximately 8 carats (diamond weight) each. These matched the weights of the pieces “cut” from the computer models. These are the heaviest pieces possible, as they could only be removed from the real stone by either cleaving or sawing with no losses from either procedure. In actuality, these pieces would be smaller, as both of these operations can only be done in specific directions in relation to the diamond crystal. However, this analysis was outside the scope of this study. It is sufficient to say that two 8 carat pieces are the biggest possible. These two pieces are very irregularly shaped, with an expected yield below 50%. Even assuming a 50% yield, the largest stones would be a maximum of four carats each. This definitively rules out the link between the Brunswick Blue and the Russian Stickpin diamonds to the Hope. If there are sister stones to the Hope resulting from the cutting of the TB to the FB, there could be two, both less than four carats. Even this is doubtful, as cleaving or sawing at the time was inexact, the orientation to the diamond crystal would have to be perfect, and there could be no loss of material from either operation.

(Modeling by Steve Attaway)
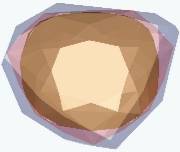

Figure 9. Three nested models (top and side views)
(Modeling by Steve Attaway)

(computer models by Steve Attaway)
Conclusion
To summarize the findings:
1. The Hope could have been cut from the French Blue.
2. There is no forensic geometric evidence prohibiting the cutting of the Hope from the French Blue.
3. The Hope model fits inside the FB model in one, and only one, way.
4. Two extra facets on the Hope could be original facets from the French Blue.
5. There were no additional significant stones from the recutting of the French Blue.
6. If there are sister-stones from the recutting of the Tavernier Blue, there are at most two, both less than four carats.
7. The Brunswick Blue and Russian Stickpin diamonds can not be sister-stones to the Hope.
Acknowledgments
Many thanks to Jeff Kaufman, a producer of the Discovery Channel, for making this all possible. The concept for this project was initially his as a subject for a Discovery Channel program concerning the Hope and its genealogy. He was also responsible for coordinating with the Smithsonian for access to the Hope for this project.
The Hope’s computer reconstruction involved the Smithsonian Institute, the current curator of the Hope. Jeffrey Post, the Gem Collections curator, Russell Feather, all of whom enthusiastically supported this project from inception to completion, as it could fill in gaps of knowledge that were historically very important. Jeff’s support was necessary to mobilize the Smithsonian so the Hope could be removed from its display and unmounted, no small feat given the security requirements. These are so stringent that the stone has been removed from its mounting just seven times since the beginning of the 20th century (as of Dec 2004). His coordination for this event took place over two months, for which we are extremely grateful.
Thanks to Nancy Attaway and Karen Sucher for their expertise in analyzing the facet patterns, and making suggestions on how to further iterate the models. Nancy also cut a cubic zirconia replica of the French Blue that is scheduled to go on display at the Smithsonian next to the Hope.
And last, but certainly not least, Steve Attaway did the yeoman’s work on the computer modeling. Figures 2, 3, 7, 8, 9, and 10 are his work.


