Specifications
Weight: 140.91 carats*
Dimensions: 30.7 x 26.1 x 19.81 mm**
Color: Yellow
Weight of Rough: Unknown
Origin: Golconda, India
Date Found: c. 1610
Current Location: Unknown
*Weight has always been reported as 137.27 carats; however, these are old carats. Weight listed here is modern carats.
**Dimensions are from measuring the replica.
Details

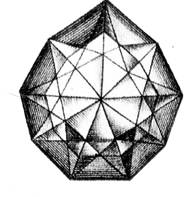
The Florentine is one of those elusive stones that is difficult to track down. Tillander (1995), p. 199, there are six line drawings of different versions of the stone as described by different sources. Tavernier has provided one of the earliest references to it in the 1600’s. Cletscher drew it in the early 1700’s from some unknown source, but it is a more rounded stone. Bauer around 1900 has his version. All three of these lived contemporaneously with the stone and may have viewed it first-hand (certainly Tavernier).Their facet patterns are similar, yet their outlines are different.
And then there is the low-resolution photograph of it in a hatpin taken around 1900, assuming it is the Florentine, that has yet a different shape and a similar facet pattern. Which to believe?
I am assuming that the photo is the real Florentine, as the shape and facet pattern bear at least some resemblance to the various line drawings. If the photo was taken around 1900, it is quite possible it is the Florentine as the stone disappeared at the close of WWI. Both the photo and all line drawings, plus descriptions given by the various authors, indicate that it is a Double Dutch Rose cut. This style of cutting uses triangular shaped facets all around, and the top and bottom of the stone are similarly faceted. All graphic references indicate this.
If the photo is considered genuine, then its outline can be used for modeling. Unfortunately, there is not enough detail in the photo to discern all the facets, but those that are visible suggest the Double Dutch Rose style. Bauer’s drawing most closely resemble the photo, but are his drawings accurate? Fortunately, he published a series of drawings of 14 other diamonds. Analysis of these stones show that he has a consistent track record for size, but the details in some are woefully lacking. Tavernier seems to be accurate in his drawing of the Great Table, as reported by Sir Harford Brydges in 1794 when he handled the stone and commented it was exactly as Tavernier described. There is evidence that Tavernier may have made some mistakes in other stones, so he has not been considered the authority in this case. Again, what to believe?
The replica described here is the result of an effort in 1981 to create it using Tavernier’s description. Although the line drawing existed in many sources, they were not considered accurate in stone dimensions due to scaling effects in printing copies of the original. It was desired to use a drawing from an original manuscript. Fortunately, one of seven in the United States existed in a university library about an hour away from where I was living.
Dimensions and facet pattern were taken from the drawing (below). Since this was before the personal computer and graphics programs, everything had to be done manually. Index settings were easy to calculate, angles were more problematic. Since the stone was described as a double Dutch rose cut, symmetrical on top and bottom, this made calculations slightly easier, as now only the top half of the stone had to be resolved.
The weight reported by Tavernier was 137.27 carats. Assuming an average specific gravity of 3.51 g/cc for diamond, the required volume for half the stone was 3.91 grams. (This was before the difference between old and new carats was known.) A side view of the stone equates to a cone of low angle sitting on top of a truncated cone of a higher angle. All that was necessary was to find the angles of the two cones.

The effort to get from this equation to determining the angle of the upper and lower cones took three years. There were many steps where the solution seemed unattainable and required considerable thought. The delay was no problem, as large CZ of the color, purity, and degree of perfection was extremely difficult to make at the time and it took that long for the manufacturer to fill the order.
The final equation to solve was 11.06=7tan s + tan y. Unfortunately, this resulted in a single equation with two unknowns; s was the angle of the lower cone and y the angle of the upper cone. This was resolved by iterating the values of s and comparing them with the resultant value of y. As it turned out, s could only range from 54-56°, as outside of this y could not be resolved. This information was used to calculate the other data necessary to cut the replica.
